Ellipse
Ellipse. Focuses. Equation of ellipse. Focal length.
Large and small axes of ellipse. Eccentricity.
Equation of tangent line to ellipse.
Tangency condition of straight line and ellipse.
An ellipse ( Fig.1 ) is called a locus of points, a sum of distances from which to the two given points F 1 and F 2 , called focuses of ellipse, is a constant value.
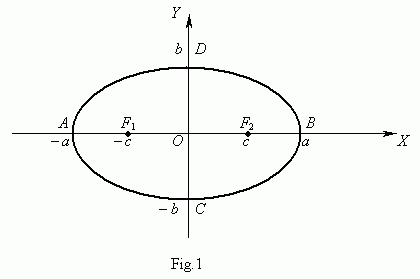
An equation of ellipse ( Fig.1 ) is :
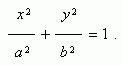
Here the origin of coordinates is a
center of symmetry of ellipse, and the coordinate axes are its axes of symmetry.
At
a
>
b
focuses of ellipse are placed on axis
ну
(
Fig.1 ), at
a
<
b
focuses of ellipse are
placed on axis
н
Y
, and at
a
=
b
an ellipse becomes a circle
( in this case focuses of
ellipse coincide with a center of circle ). Thus,
a circle
is a particular case of an
ellipse
.
The segment
F
1
F
2
= 2
Я
,
where
is called
a
focal length
. The
segment
AB
= 2
a
is called
a
large axis
of
ellipse, the segment
CD
= 2
b
is called
a small axis
of ellipse. The number
e
=
c
/
a
,
e
< 1 is called
an eccentricity
of ellipse.
Let п ( У 1 , С 1 ) be a point of ellipse, then an equation of tangent line to ellipse in this point is
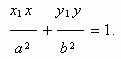
A tangency condition of a straight line y = m x + k and an ellipse У 2 / a 2 + С 2 / b 2 = 1 :
k 2 = m 2 a 2 + b 2 .